A capacitor stores electrical energy differently from batteries. It redistributes electrons without producing them. When connected to a battery, it charges and stores energy for use. A basic capacitor, like a parallel plate capacitor, consists of two metal plates separated by a gap with an insulator, typically air, in between.
. 
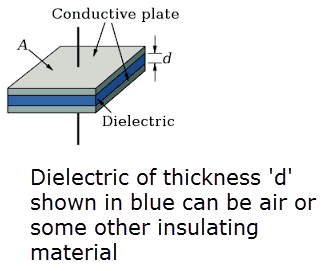
Figure 1 - Parallel plate capacitor
In Figure 1, connecting the top plate to the battery's positive terminal and the bottom plate to the negative terminal charges the capacitor. Electrons move, creating positive and negative charges on the plates. This makes a brief light bulb glow when connected, but it turns off when the plates regain neutrality.
Capacitors are rated by their capacitance, measured in Farads or Microfarads. More capacitance means the capacitor can store more charge.
The dielectric in a capacitor is crucial because it affects the electric field within it. As the capacitor charges, the electric field builds up in the dielectric. However, if the electric field becomes too strong, the dielectric can break down and turn into a conductor, rendering the capacitor unable to store charge.
Let's consider a scenario with multiple dielectrics in a capacitor, as depicted in Figure 2. Our goal is to calculate the capacitance of this capacitor using EMS.
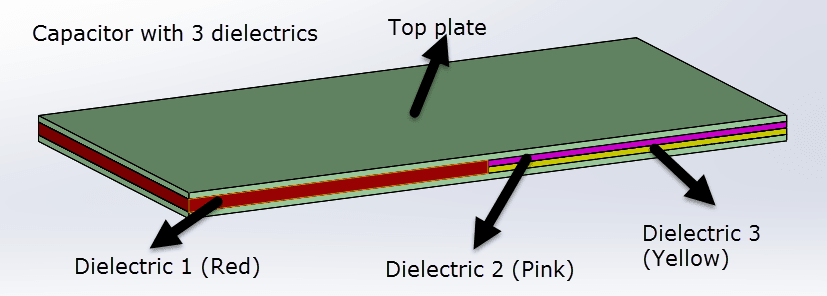
Figure 2 - Capacitor with 3 dielectrics
EMS has the capability to:
Calculate the capacitance of the capacitor with multiple dielectrics.
Compute the electric field within the dielectric region.
Predict the possibility of a breakdown occurring in the capacitor.
According to EMS, the capacitance value is 6e-11 Farads, as shown in Figure 3. Additionally, Figure 4 displays the electric field distribution in the dielectric region when the capacitor is connected to a 12 V battery.
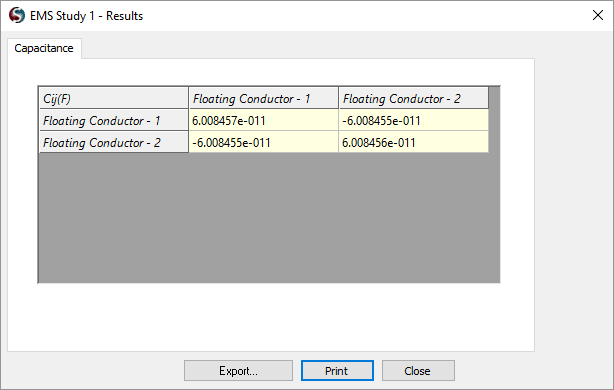
Figure 3 - Capacitance as computed by EMS
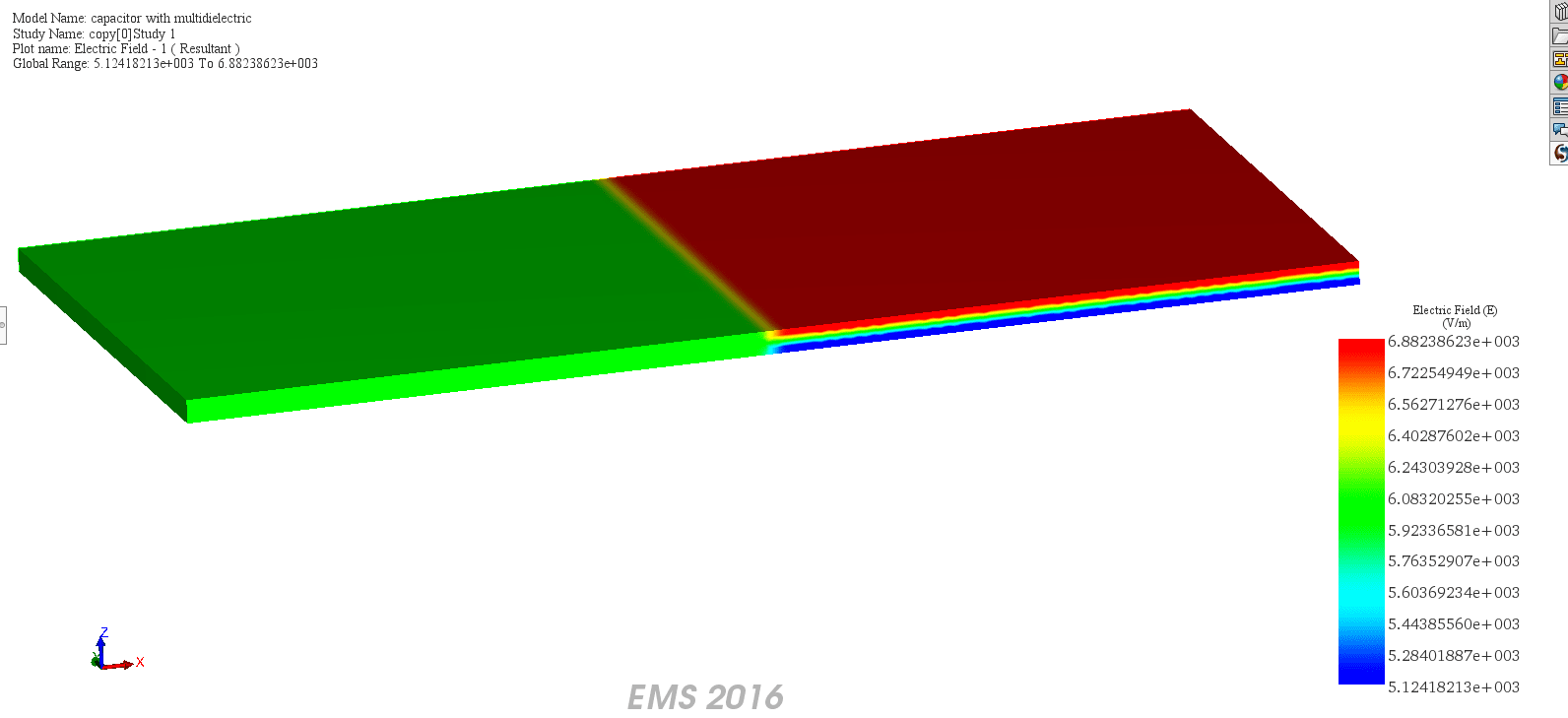
Figure 4 - Electric field distribution in the dielectirc
| Theoretical Result | EMS Result | |
| Capacitance (F) | 60.08e -12 F | 60.084e -12 F |
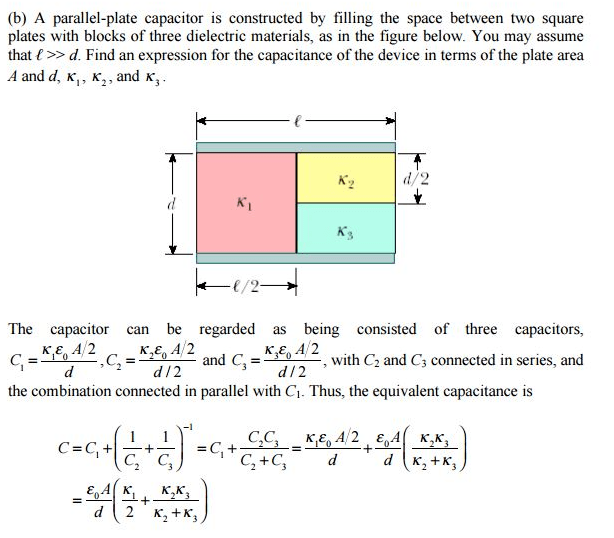
The formula for the theoretical result is derived from the source mentioned below.
This application note elucidates the fundamentals of capacitors, their mechanism for storing charge, and the crucial role of the dielectric material. It differentiates capacitors from batteries by highlighting their ability to redistribute electrons rather than produce them, thus storing electrical energy for immediate use. The note further delves into how capacitors are measured in terms of capacitance, with higher capacitance indicating a greater charge storage capacity. A significant focus is placed on the dielectric's function, emphasizing its importance in maintaining the capacitor's ability to store charge without succumbing to breakdown under strong electric fields.
An example featuring a capacitor with three different dielectrics is presented to demonstrate EMS software's capability to calculate capacitance accurately. EMS not only computes the capacitance, which aligns closely with theoretical results but also visualizes the electric field distribution within the dielectric, thereby assessing the risk of dielectric breakdown. This application note showcases the practical application of EMS in evaluating capacitor designs, ensuring their reliability and effectiveness in various electrical circuits and systems.
MIT OpenCourseWare - http://ocw.mit.edu (8.02SC Physics II: Electricity and Magnetism, Fall 2010)
| Share on |
English (CA)
Deutsch
日本語