Exploring the Behavior of Eddy Currents in a Conducting Disc

Physics 

A cylindrical region contains a homogenous, time-varying magnetic field  ; B₀ is the field magnitude and  is vertical unit vector. Since there is no electric charge in the system, the changing magnetic field is the sole cause of the electric field  . According to Farady's equation :

     (eq.1)

or in the case where  only has z component:
 

       (eq.2)

where  r and   represent radial and angular coordinates of the cylindrical coordinate system (Fig. 1).

Due to the symmetry of the problem, its analysis can be simplified by noting that  does not depend on , i.e.  . Equation 2 therefore, takes the following form:

      (eq.3)

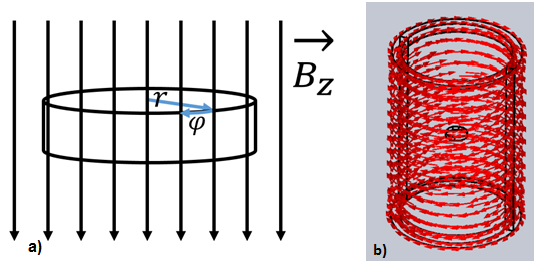
Figure 1 - a) Copper disc in vertical magnetic field;b) Changing magnetic field is produced inside a cylinder wrapped in coils that carry AC current;
 

To solve for  , equation 3 is multiplied by  and then integrated from 0 to  as:

                                         

or  

If a copper disc is placed inside the cylinder, as a consequence of the induced electric field, eddy currents are distributed inside the disc. Current density  is, according to the Ohm’s law :

where  is specific conductivity of copper ( ). For a magnetic field with a magnitude of

 and angular frequency , magnitude of  current density is  . Induced eddy currents lag the change in flux density by 90^º.

Model

Eddy current distribution in a copper disc can be easily simulated in EMS as a AC Magnetic  study. To create a uniform magnetic field inside the cylinder, allow a certain thickness to its wall so that you can define the wall as a wound coil. A cylinder with inner radius of 15mm and height of 50mm, should be used to define a Wound Coil with 100 turns and RMS current magnitude per turn of .For the Current phase select 0º - this will produce a cosine current profile in the coil. This current will in turn induce a relatively uniform flux density of magnitude  over the volume of the copper disc (radius: 3mm), placed in the center of the cylinder. To help magnetic field align vertically inside the cylinder, add Normal Flux boundary conditions to cylinder caps and Tangential Flux boundary condition to the inner face of the cylinder wall. 

Boundary conditions

1. In the EMS manger tree right-click on the Load/Restraint  folder and select Normal Flux .
2. Click inside the Faces for Normal Flux box  then select Cylinder caps .
3. Click OK .

1. In the EMS manger tree right-click on the Load/Restraint  folder and select Tangential Flux .
2. Click inside the Faces for Tangential Flux box  then select the inner face of the Cylinder wall .  
3. Click OK .

Coils

Eddy Effects

To set up Eddy Effects, right-click the copper disc in the EMS tree and select Turn on Eddy Effects  .

Meshing

To get a high resolution of current density results in the copper disc, a Mesh control of 0.1 mm should be applied to the copper disc.
To do so,

1. In the EMS manger tree right-click on the Mesh  folder and select Apply Mesh Control .
2. Click inside the Bodies box then select the inner face of the copper disc .  
3. Under Control Parameters click inside the Element Size box and type 0.1 mm.
4. Click OK .

To mesh the model:

1. In the EMS manger tree, right-click on the Mesh icon and select Create Mesh  .
2. Click OK  .

Results

Current Density

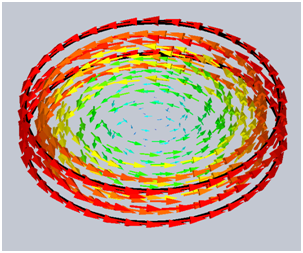
Figure 2 - 3D vector plot of Eddy current density inside the copper disc

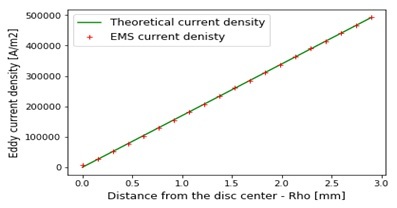
Figure 3 - Comparison of EMS and theoretical results for eddy current density
 

The agreement between the theoretical solution () and the EMS 2D current density plot is displayed in Figure 3. 
 

Conclusion